I was recently at the awesome Beaker Street public science festival in Hobart, presenting with the likes of national treasure Dr Karl, the ABC’s Robyn Williams, Aussie astronaut Katherine Bennell-Pegg, and Dr Tiana Pirtle who gave an amazing talk on the science of animal vaginas. #NerdHeaven
What the fact (orial)?!
Thanks to those who came along to my Beaker Street talk “Numb and Number”, where I explored the beautiful mathematics of factorials. (I also blew everyone’s mind with infinite infinities, but that’s for another newsletter. 😊)
Factorials occur all the time in mathematics, especially in probability and counting problems. And 24 is one of the most fascinating factorials of them all.
First, what's a factorial? If I was stacking 4 different books in a pile, they can be stacked 4 x 3 x 2 x 1 = 24 different ways. Because 24 = 4 x 3 x 2 x 1, we call it 4 ‘factorial’ or 4!
Factorials grow incredibly quickly. For example:
22! = 1,124,000,727,777,607,680,000 which has exactly 22 digits.
23! = 25,852,016,738,884,976,640,000 which has exactly 23 digits.
And 24! = 620,448,401,733,239,439,360,000 is the final factorial where the answer has the exact same number of digits!!!
How cool is that!!!
The fourth dimension
We live in a world with three dimensions – or 3D – of space, and we can picture things that are 3D, 2D and 1D fairly easily. For example, we can see a box with width, depth and height is 3D. We can see a piece of paper with no depth at all is 2D. We can see a thin straight wire without much height or depth as a 1D straight line.
But when it comes to picturing objects that exist in four dimensions (4D) of space, we understandably struggle.
In the same way that four straight lines can be used to make a 2D square, and six 2D squares can form a 3D cube, in 4D space we can use eight 3D cubes to assemble the next shape in this series, which we call a ‘tesseract’ or ‘4D hypercube’.
While this nifty diagram won’t help you spot a tesseract at the shops, it will help you count its many elements - eight cubes (don’t forget about the inner and outer cubes!), 24 faces, 32 edges, and 16 corners or vertices.
Fascinating fast facts about 24!
B-B-B-B-Barbarino
Backgammon is played with great passion around the world on a board with 24 points. Cool backgammon terms include ‘lover’s leap’ (an opening roll of 6 and 5); ‘anti-joker’ (a very bad roll), and a ‘Barabino’ (a roll of 5-4 from the bar used to make the opponent’s five-point). Huh?
Fly me to the moon
Between 1968-1972, twenty-four American astronauts made the trip from Earth to the Moon. Three made the journey twice – James Lovell (Apollo 8, Apollo 13), John Young (Apollo 10, Apollo 16), and Gene Cernan (Apollo 10, Apollo 17) who famously took what is currently our last-ever step on the moon on 14 December 1972.
24 (horny) rabbits
In 1859 Geelong farmer Thomas Austin released Australia’s first-ever rabbits – 24 in total – onto his property. A member of the VIC Acclimatisation Society, he imported many species into Australia, including blackbirds and thrushes, with reports the rabbits were intended as game for shooting parties. Hey Tom, either your mates were terrible shots or you should have thought things through a bit more as there are now an estimated 200 million rabbits in Australia!
The game of 24
If you’re looking for a great card game to get your primary school child crunching out the arithmetic, you could do a lot worse than the card game 24.
Each card in the deck has 4 numbers. For older ages, the cards feature whole numbers, fractions and decimals.
The challenge is to use all 4 numbers on the card once, and once only, to get an answer of 24. For example, for the card shown:
5 x 10 = 50, 50 x .5 = 25 and 25 - 1 = 24
The reason the number 24 is chosen for this game is because its many factors 1,2,3,4,6,8 and 12 allow multiple possibilities for correct answers. Check out 24game.com
TRIVIA: Win an Adam Spencer maths book!
I’ll give a signed copy of one of my books to a couple of readers who crack this beast of a puzzle...
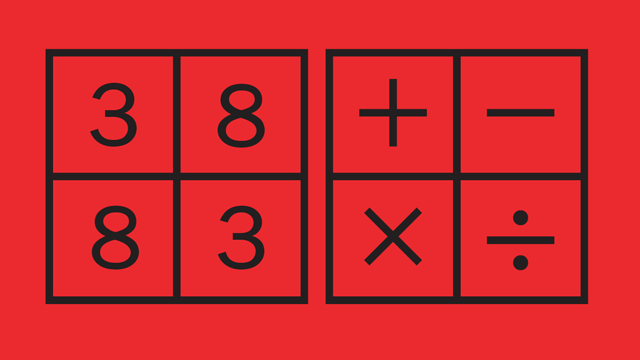
Using the four numbers 3, 3, 8, and 8, and any of the usual four arithmetic operations (addition, subtraction, multiplication and division), can you make the number 24?
‘Ha! Adam, you goose,’ you reply, ‘that’s easy. 3 x 8 = 24. I didn’t even need one of your 3s and one of the 8s.’ Your smile holds for about as long as it takes me to say, ‘No, you have to use all four numbers — both 3s and both 8s.’
No tricks are needed. You don’t need to join 8 and 3 to make 83, or raise numbers to powers or take square roots or factorials or anything else of that kind. Just plus, minus, multiplication and division. ‘Back at ya, playa!’
You can use fractions on the way, of course, because they are the same as division. But the final answer must be exactly 24. For example: 3 x 8 + (3/8) makes 24 and three-eighths, which is quite close, but no banana.
Trust me ... this one is really tough. Good luck! I will send a signed copy of my book to three (3) randomly chosen readers who correctly answer the puzzle. Send your answer via email here by Sunday 18th August, 5pm AEST.
Answer to last issue's trivia
I was flooded with answers to my last brainbuster. Did you get it right? The answer is 38!
The clock shows 2 o’clock, so that value is 2 (unlike the clocks above showing 3 o'clock). There are 3 bananas in each bunch, so those values are 3 (unlike the bunches above which have 4). Finally, the shape has 11 edges (it’s missing the square), so that value is 11. Leaving us with 2 + 3 + 3 x 11.
Next, did you remember the order of operations? 2 + 3 + [3 x 11] = 38
The lucky winners - Aaron Cowper, Natalie Wee and David Bailey - have each received a signed copy of a maths book of mine. Enjoy!!
That’s all from me for now. If you'd like more geeky fun, please check out my other newsletters below, or connect with me on LinkedIn and/or X.
Yours in numbers,
Adam
Jump back to: Fun factorial 4D Bararino Astronauts Rabbits Game24 Competition Answer